

In this section we will consider two more cases where it is possible to conclude that triangles are congruent with only partial information about their sides and angles, Suppose we are told that \(\triangle ABC\) has \(\angle A = 30^, \angle B = 40^\), and \(AB =\) 2 inches. Let us attempt to sketch \(\triangle ABC\). We first draw a line segment of 2 inches and label it \(AB\), With a protractor we draw an angle of \(30^\) at \(A\) and an angle of \(40^\) at \(B\) (Figure \(\PageIndex\)). We extend the lines forming \(\angle A\) and \(\angle B\) until they meet at \(C\). We could now measure \(AC, BC\), and \(\angle C\) to find the remaining parts of the triangle. 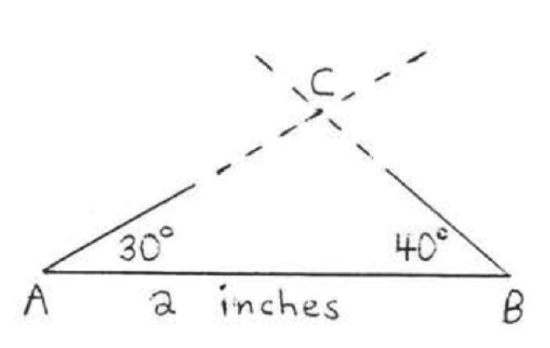
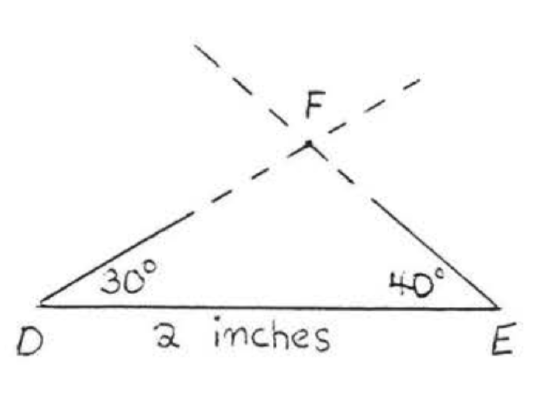
Figure \(\PageIndex<1>\): (left) Sketching \(\triangle ABC\) and (right) Sketching \(\triangle DEF\). Let \(\triangle DEF\) be another triangle, with \(\angle D = 30^\), \(\angle E = 40^\), and \(DE =\) 2 inches. We could sketch \(\triangle DEF\) just as we did \(\triangle ABC\), and then measure \(DF, EF\), and \(\angle F\) (Figure \(\PageIndex\)). It is clear that we must have \(AC = DF\), \(BC = EF\), and \(\angle C = \angle F\), because both triangles were drawn in exactly the same way, Therefore \(\triangle ABC \cong \triangle DEF\). In \(\triangle ABC\) we say that \(AB\) is the side included between \(\angle A\) and \(\angle B\). In \(\triangle DEF\) we would say that DE is the side included between \(\angle D\) and \(\angle E\). Our discussion suggests the following theorem:
Two triangles are congruent if two angles and an included side of one are equal respectively to two angles and an included side of the other.
In Figure \(\PageIndex<1>\) and \(\PageIndex\), \(\triangle ABC \cong \triangle DEF\) because \(\angle A, \angle B\), and \(AB\) are equal respectively to \(\angle D\), \(\angle E\), and \(DE\). We sometimes abbreviate Theorem \(\PageIndex<1>\) by simply writing \(ASA = ASA\).
Solution
Note that the included side is named by the two letters representing each of the angles. Therefore, for (1), the side included between \(\angle P\) and \(\angle Q\) is named by the letters \(P\) and \(Q\) -- that is, side \(PQ\). Similarly for (2) and (3).
For the two triangles in the diagram
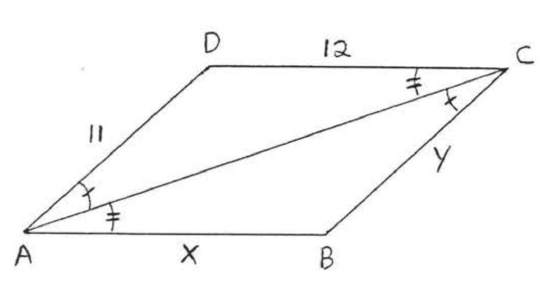
Solution
(1) From the diagram \(\angle A\) in \(\triangle ABC\) is equal to \(\angle C\) in \(\triangle ADC\). Therefore, "\(A\)" corresponds to "\(C\)". Also \(\angle C\) in \(\triangle ABC\) is equal to \(\angle A\) in \(\triangle ADC\). So "\(C\)" corresponds to "\(A\)". We have

(2) \(\angle A, \angle C\), and included side \(AC\) of \(\triangle ABC\) are equal respectively to \(\angle C\), \(\angle A\), and included side \(CA\) of \(\triangle CDA\). (\(AC = CA\) because they are just different names for the identical line segment, We sometimes say \(AC = CA\) because of identity.) Therefore \(\triangle ABC \cong \triangle CDA\) because of the ASA Theorem (\(ASA = ASA\)).
Summary:
(3) \(AB = CD\) and \(BC = DA\) because they are corresponding sides of the congruent triangles. Therefore \(x = AB = CD = 12\) and \(y = BC = DA = 11\).
Answer:
(1) \(\triangle ABC \cong \triangle CDA\).
(2). \(ASA = ASA\): \(\angle A, AC, \angle C\) of \(\triangle ABC = \angle C\), \(CA\), \(\angle A\) of \(\triangle CDA\).
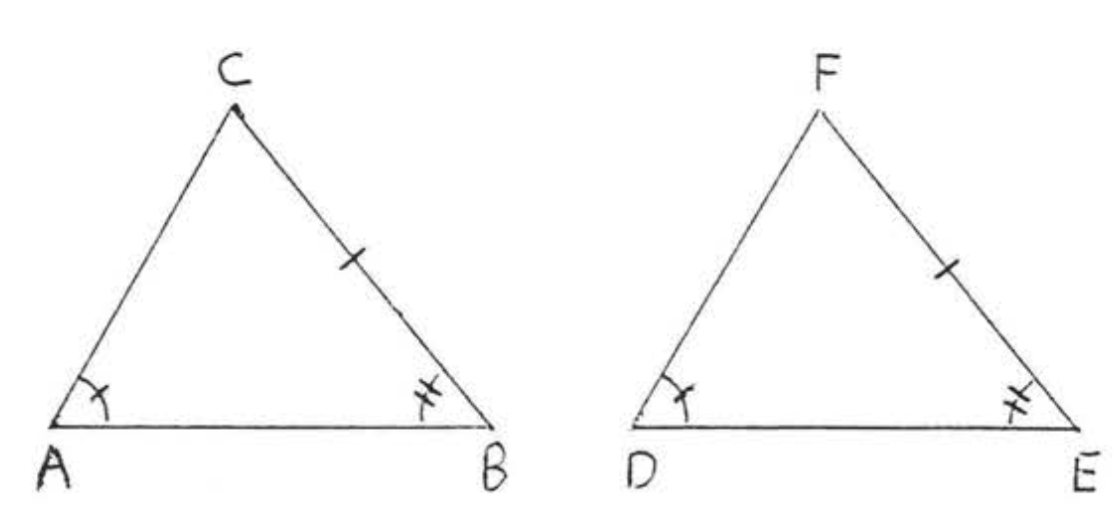
Let us now consider \(\triangle ABC\) and \(\triangle DEF\) in Figure \(\PageIndex\). \(\angle A\) and \(\angle B\)
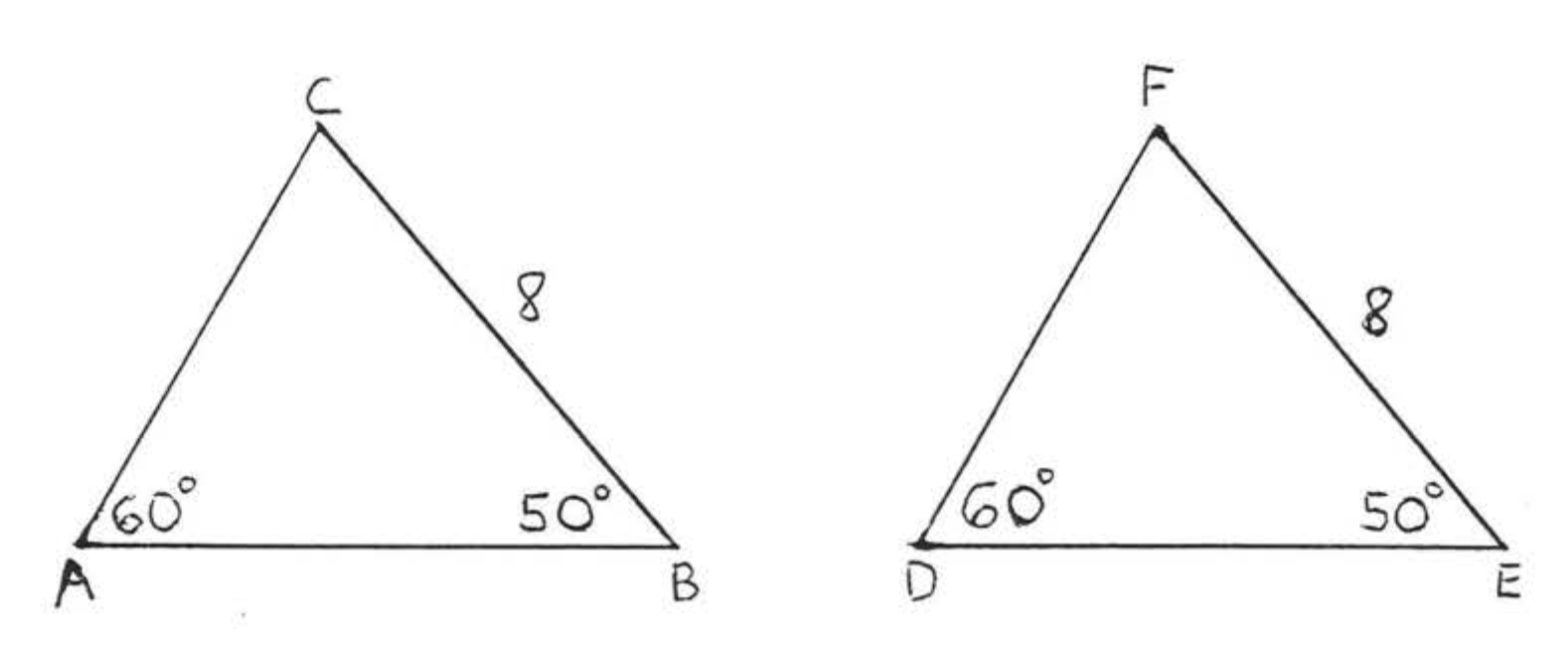
of \(\triangle ABC\) are equal respectively to \(\angle D\) and \(\angle E\) of \(\triangle DEF\), yet we have no information about the sides included between these angles, \(AB\) and \(DE\), Instead we know that the unincluded side BC is equal to the corresponding unincluded side \(EF\). Therefore, as things stand, we cannot use \(ASA = ASA\) to conclude that the triangles are congruent, However we may show \(\angle C\) equals \(\angle F\) as in Theorem \(\PageIndex\), section 1.5 \((\angle C = 180^ - (60^ + 50^) = 180^ - 110^ = 70^\) and \(\angle F = 180^ - (60^ + 50^) = 180^ - 110^ = 70^)\). Then we can apply the ASA Theorem to angles Band \(C\) and their included side \(BC\) and the corresponding angles \(E\) and \(F\) with included side EF. These remarks lead us to the following theorem:
Two triangles are congruent if two angles and an unincluded side of one triangle are equal respectively to two angles and the corresponding unincluded side of the other triangle (\(AAS = AAS\)).
In Figure \(\PageIndex\), if \(\angle A = \angle D\), \(\angle B = \angle E\) and \(BC = EF\) then \(\triangle ABC \cong \triangle DEF\).
Proof
\(\angle C = 180^ - (\angle A + \angle B) = 180^ - (\angle D + \angle E) = \angle F\). The triangles are then congruent by \(ASA = ASA\) applied to \(\angle B\). \(\angle C\) and \(BC\) of \(\angle ABC\) and \(\angle E, \angle F\) and \(EF\) of \(\triangle DEF\).
For two triangles in the diagram

Solution
(1) \(\triangle ACD \cong \triangle BCD\).
(2) \(AAS = AAS\) since \(\angle A, \angle C\) and unincluded side \(CD\) of \(\angle ACD\) are equal respectively to \(\angle B, \angle C\) and unincluded side \(CD\) of \(\triangle BCD\).
(3) \(AC = BC\) and \(AD = BD\) since they are corresponding sides of the congruent triangles. Therefore \(x = AC = BC = 10\) and \(y = AD = BD\). Since \(AB = AD + BD = y + y = 2y = 12\), we must have \(y = 6\).
Answer
(1) \(\triangle ACD \cong \triangle BCD\)
(2) \(AAS = AAS\): \(\angle A, \angle C, CD\) of \(\triangle ACD = \angle B, \angle C, CD\) of \(\triangle BCD\).
For the two triangles in the diagram
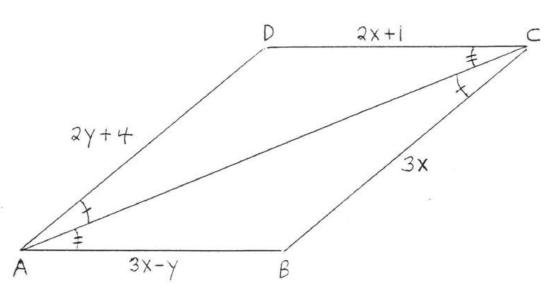
Solution
Part (1) and part (2) are identical to Example \(\PageIndex\).
We solve these equations simultaneously for \(x\) and \(y\):
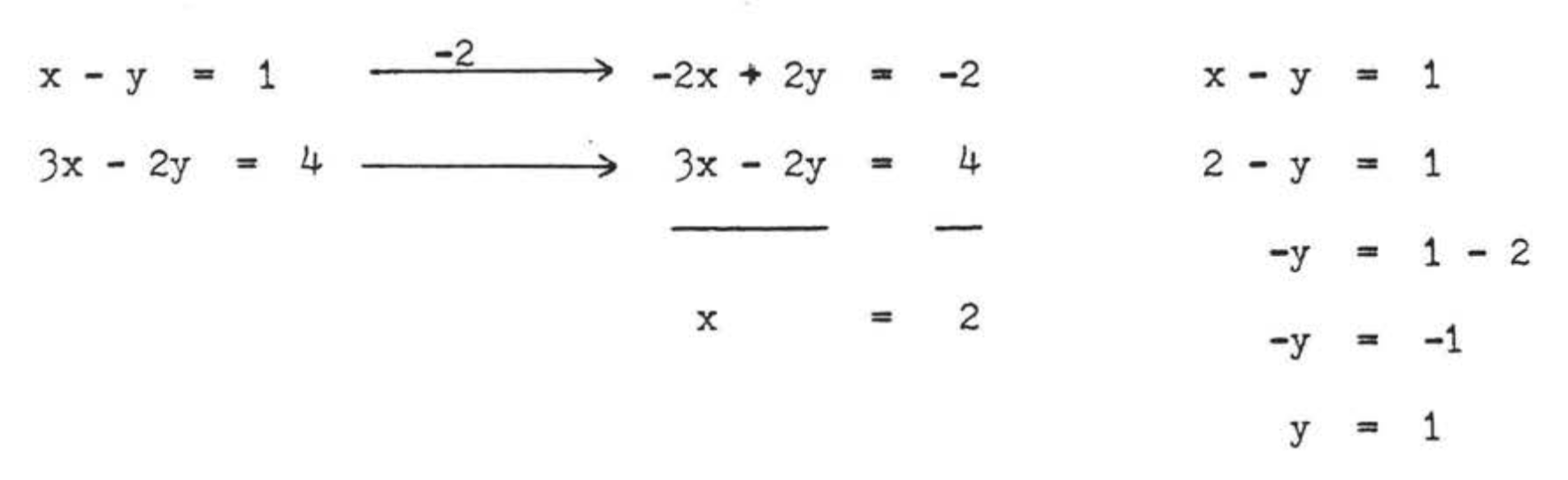

(1) and (2) same as Example \(\PageIndex\).
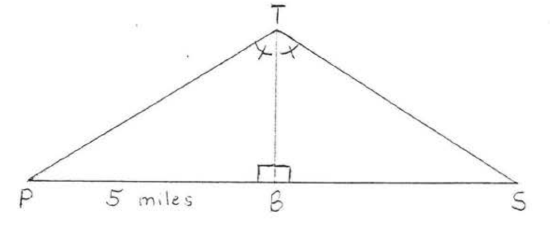
From the top of a tower Ton the shore, a ship Sis sighted at sea, A point \(P\) along the coast is also sighted from \(T\) so that \(\angle PTB = \angle STB\). If the distance from \(P\) to the base of the tower \(B\) is 3 miles, how far is the ship from point Bon the shore?

Solution
\(\triangle PTB \cong \triangle STB\) by \(ASA = ASA\). Therefore \(x = SB = FB = 3\).
Answer: 3 miles
The method of finding the distance of ships at sea described in Example \(\PageIndex\) has been attributed to the Greek philosopher Thales (c. 600 B.C.). We know from various authors that the ASA Theorem has been used to measure distances since ancient times, There is a story that one of Napoleon's officers used the ASA Theorem to measure the width of a river his army had to cross, (see Problem 25 below.)
1 - 4. For each of the following (1) draw the triangle with the two angles and the included side and (2) measure the remaining sides and angle,
1. \(\triangle ABC\) with \(\angle A = 40^\), \(\angle B = 50^\), and \(AB = 3\) inches,
2. \(\triangle DEF\) with \(\angle D = 40^\), \(\angle E = 50^\), and \(DE = 3\) inches,
3. \(\triangle ABC\) with \(\angle A = 50^\), \(\angle B = 40^\), and \(AB = 3\) inches,
4. \(\triangle DEF\) with \(\angle D = 50^\), \(\angle E = 40^\), and \(DE = 3\) inches.
5 - 8. Name the side included between the angles:
5. \(\angle A\) and \(\angle B\) in \(\triangle ABC\).
6. \(\angle X\) and \(\angle Y\) in \(\triangle XYZ\).
7. \(\angle D\) and \(\angle F\) in \(\triangle DEF\).
8. \(\angle S\) and \(\angle T\) in \(\triangle RST\).
9 - 22. For each of the following
(1) write a congruence statement for the two triangles,
(2) give a reason for (1) (SAS, ASA, or AAS Theorems),
(3) find \(x\), or \(x\) and \(y\).
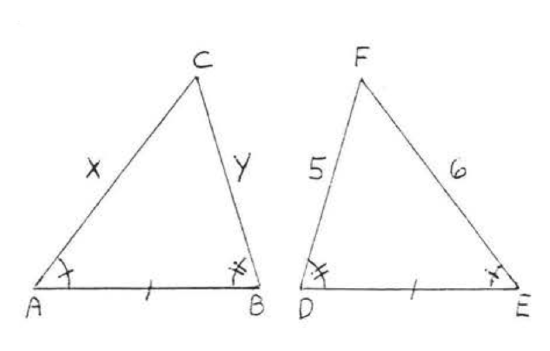
9. 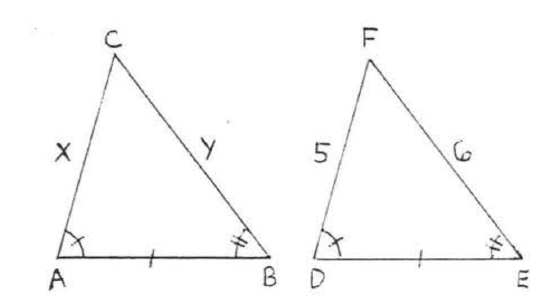 10.
10. 
11. 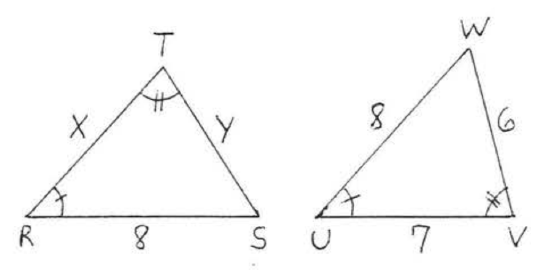 12.
12. 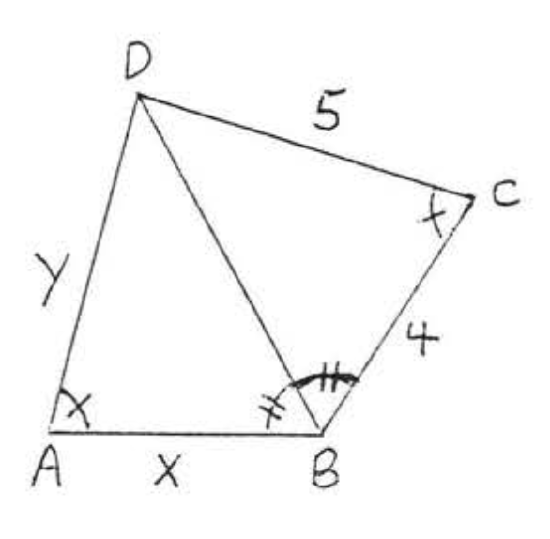
13.  14.
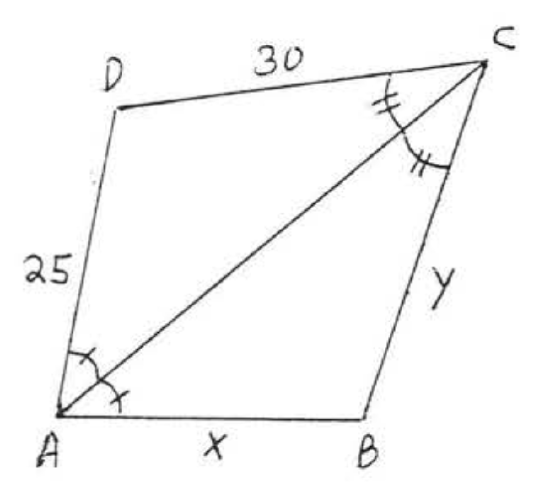
14. 
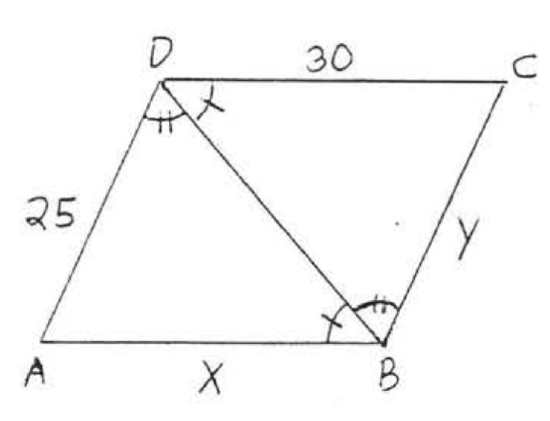
15.  16.
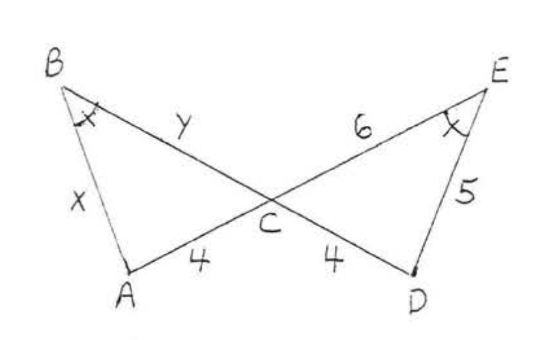
16. 
17. 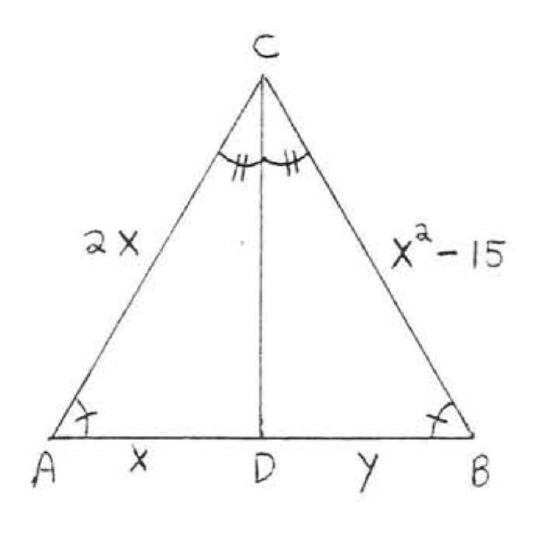 18.
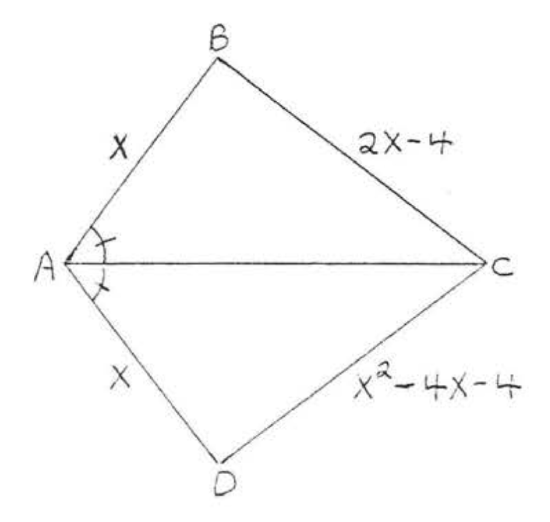
18. 
19.  20.
20. 
21. 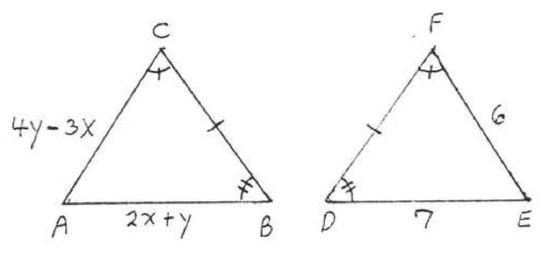 22.
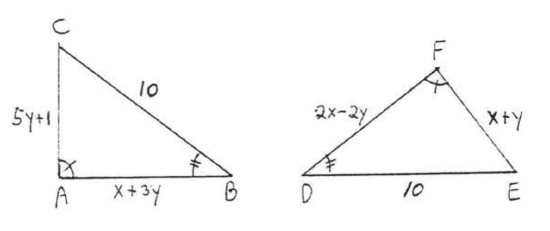
22. 
23 - 26. For each of the following, include the congruence statement and the reason as part of your answer:
23. In the diagram how far is the ship S from the point \(P\) on the coast?
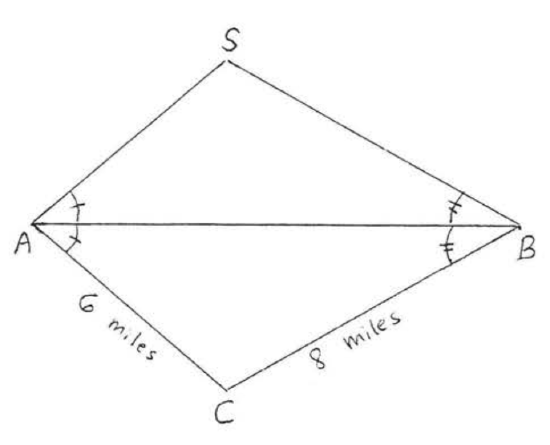
24. Ship \(S\) is observed from points \(A\) and \(B\) along the coast. Triangle \(ABC\) is then constructed and measured as in the diagram, How far is the ship from point \(A\)?
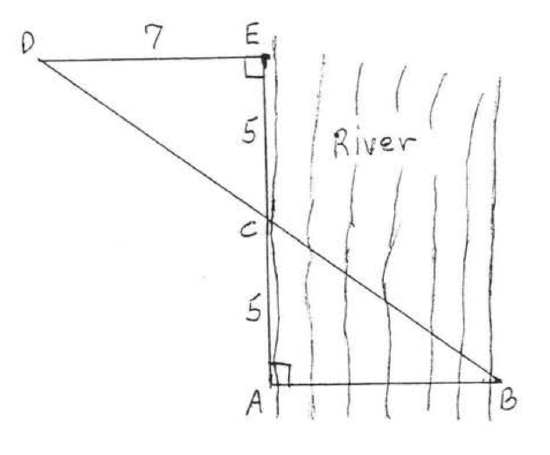
25. Find the distance \(AB\) across a river if \(AC = CD = 5\) and \(DE = 7\) as in the diagram.
26. that is the distance across the pond?

This page titled 2.3: The ASA and AAS Theorems is shared under a CC BY-NC-SA 4.0 license and was authored, remixed, and/or curated by Henry Africk (New York City College of Technology at CUNY Academic Works) via source content that was edited to the style and standards of the LibreTexts platform.